
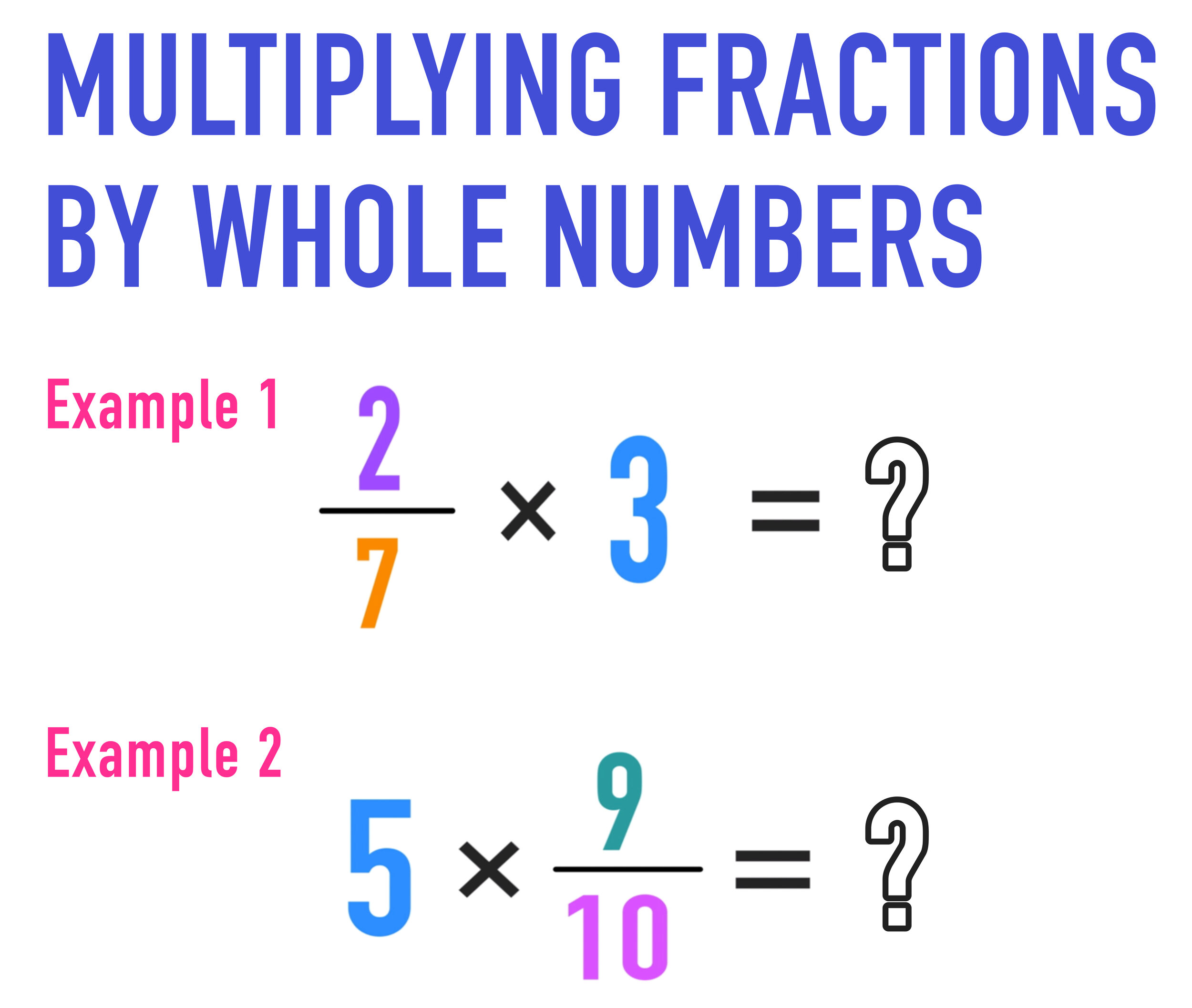
When we arrive at 50 as the remainder, and bring down the "0", we find ourselves dividing 500 by 74, which is the same problem we began with. Observe that at each step we have a remainder the successive remainders displayed above are 56, 42, 50. For example, consider the rational number 5 / 74:Įtc.

In order to convert a rational number represented as a fraction into decimal form, one may use long division. For example, 1.2 34 may be read "one point two repeating three four", "one point two repeated three four", "one point two recurring three four", "one point two repetend three four" or "one point two into infinity three four".ĭecimal expansion and recurrence sequence In English, there are various ways to read repeating decimals aloud. This notation introduces uncertainty as to which digits should be repeated and even whether repetition is occurring at all, since such ellipses are also employed for irrational numbers π, for example, can be represented as 3.14159.Ġ. Informally, repeating decimals are often represented by an ellipsis (three periods, 0.333.), especially when the previous notational conventions are first taught in school.(See examples in table below, column Arc.) In Spain and some Latin American countries, the arc notation over the repetend is also used as an alternative to the vinculum and the dots notation.(See examples in table below, column Parentheses.) This can cause confusion with the notation for standard uncertainty. In parts of Europe, Vietnam and Russia, the convention is to enclose the repetend in parentheses.(See examples in table below, column Dots.) In the United Kingdom, New Zealand, Australia, India, South Korea, and mainland China, the convention is to place dots above the outermost numerals of the repetend.(See examples in table below, column Vinculum.) In the United States, Canada, India, France, Germany, Switzerland, Czechia, and Slovakia the convention is to draw a horizontal line (a vinculum) above the repetend.There are several notational conventions for representing repeating decimals. 8 Multiplication and cyclic permutation.
#1 3 times 1 3 fraction series#
7 Repeating decimals as infinite series.6 Converting repeating decimals to fractions.5 Reciprocals of integers not coprime to 10.4 Reciprocals of composite integers coprime to 10.1.4 Every repeating or terminating decimal is a rational number.1.3 Every rational number is either a terminating or repeating decimal.


 0 kommentar(er)
0 kommentar(er)
